The zoo plot meets the swampland: mutual (in)consistency of single-field inflation, string conjectures, and cosmological data

Abstract

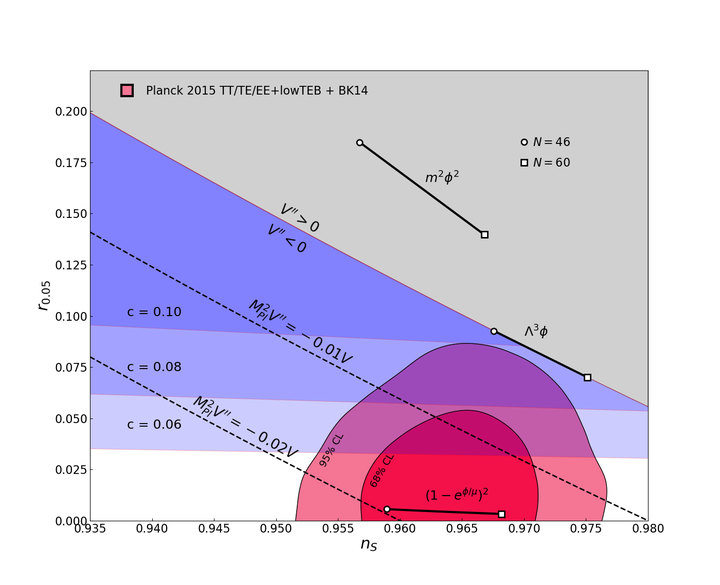
We consider single-field inflation in light of string-motivated swampland conjectures suggesting that effective scalar field theories with a consistent UV completion must have field excursion $\Delta \phi \lesssim M_{\rm Pl}$, in combination with a sufficiently steep potential, $M_{\rm Pl} V_\phi/V \gtrsim {\cal O}(1)$. Here, we show that the swampland conjectures are inconsistent with existing observational constraints on single-field inflation. Focusing on the observationally favoured class of concave potentials, we map the allowed swampland region onto the $n_S$-$r$ zoo plot of inflationary models, and find that consistency with the Planck satellite and BICEP2/Keck Array requires $M_{\rm Pl} V_\phi/V \lesssim 0.1$ and $-0.02 \lesssim M_{\rm Pl}^2 V_{\phi\phi}/V < 0$, in strong tension with swampland conjectures. Extension to non-canonical models such as DBI Inflation does not significantly weaken the bound.

Luca Visinelli

Tenure-track Associate Professor, Shanghai Jiao Tong University; Fellow, Tsung-Dao Lee Institute

PhD in Physics, working on Particle Astrophysics and Dark Matter modeling.